Emissivity and absorption coefficients
shown in §2.10.1 are related to
the Einstein's coefficients in §2.10.2.
Net absorption is defined as the absorption minus the induced emission. Energy absorbed in unit volume is expressed as follows:
integrating equation (2.123) over the solid angle,
the first term of the rhs becomes
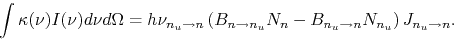 |
(2.139) |
Assuming isotropic radiation field, since
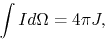 |
(2.140) |
the absorption coefficient integrated over the frequency range
of one transition is written as
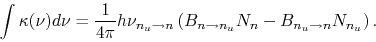 |
(2.141) |
As for the emission, the second term of equation (2.123)
becomes
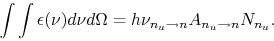 |
(2.142) |
Thus,
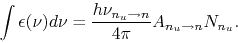 |
(2.143) |
Subsections
Kohji Tomisaka
2009-12-10