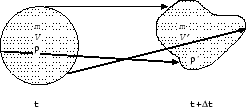 |
Consider a fluid element with mass ![]() as in A.1.
From the Newton's second law of mechanics,
the acceleration of an element is written as
as in A.1.
From the Newton's second law of mechanics,
the acceleration of an element is written as
In fluid dynamics,
using the mass density ![]() and the force working on the unit volume
and the force working on the unit volume
![]() equation (A.1) is rewritten as
equation (A.1) is rewritten as
Which kind of force works in a fluid?
Gas pressure force does work in any fluids.
Beside this, if there is the gravity,
![]() should be included in
should be included in
![]() .
If the electric currents is running in the fluid and the magnetic fields exist,
the Lorentz force
.
If the electric currents is running in the fluid and the magnetic fields exist,
the Lorentz force
![]() should be added.
should be added.
To write down the expression of the gas pressure force,
consider a fluid element between ![]() and
and ![]() as shown in Figure A.2.
Pressure force exerting on the surface
as shown in Figure A.2.
Pressure force exerting on the surface ![]() at
at ![]() is
is ![]() , while
that on the opposite side is
, while
that on the opposite side is
![]() .
The net pressure force working on the volume of
.
The net pressure force working on the volume of ![]() is equal to
is equal to
![]() , which is approximated as
, which is approximated as
![]() ).
).