


Next: The Poisson Equation of
Up: Physical Background
Previous: Physical Background
Contents
The basic equation of hydrodynamics are (1) the continuity equation of the density
[equation (A.11)],
 |
(2.1) |
(2) the equation of motion [equation (A.7)]
![\begin{displaymath}
\rho \left[ \frac{\partial {\bf v}}{\partial t}+\left({\bf v}\cdot \nabla\right) {\bf v} \right]=-\nabla p +\rho {\bf g},
\end{displaymath}](img299.png) |
(2.2) |
and (3) the equation of energy [equation (A.23)]
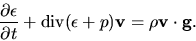 |
(2.3) |
Occasionally barotropic relation 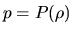 substitutes the energy equation (2.3).
Especially polytropic relation
substitutes the energy equation (2.3).
Especially polytropic relation
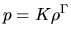 is often used on behalf of the energy equation.
In the case that the gas is isothermal or isentropic,
the polytropic relations of
is often used on behalf of the energy equation.
In the case that the gas is isothermal or isentropic,
the polytropic relations of
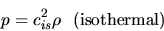 |
(2.4) |
and
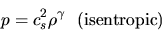 |
(2.5) |
are substitution to equation (2.3).
[We can replace equation (2.3) with equations (2.4) and
(2.5).]



Next: The Poisson Equation of
Up: Physical Background
Previous: Physical Background
Contents
Kohji Tomisaka
2007-11-02
![]() substitutes the energy equation (2.3).
Especially polytropic relation
substitutes the energy equation (2.3).
Especially polytropic relation
![]() is often used on behalf of the energy equation.
In the case that the gas is isothermal or isentropic,
the polytropic relations of
is often used on behalf of the energy equation.
In the case that the gas is isothermal or isentropic,
the polytropic relations of